Print this

Finding the Cutoff Frequency

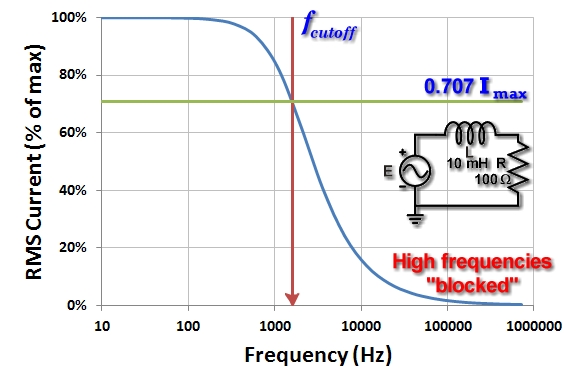
Figure 1. Frequency response of a series RL circuit

AC circuits with capacitors and inductors respond to the frequency, as well as the voltage. We can find the frequency value that reduces the circuit power to half the maximum.

Frequency response in series RL circuits

The magnetic field within an inductor L resists the rapid changes of AC signals. Slow changes, like those occurring in low-frequency signals, pass through inductors unimpeded, but rapidly changing, high-frequency signals are "resisted" by the inductor. That means less current at high frequencies, so less power delivered to the series resistor R. Figure 1 shows the drop in current as the frequency increases in a series RL circuit.

The cutoff frequency f_c is an important parameter to describe this behavior. This is the frequency at which the power delivered by the circuit is cut in half. Since P = I²R, half the power will occur when the current drops to I_max, or about 0.707 I_max. In your SPARKS circuits, you can measure the voltage drop V_R across the resistor as you adjust the frequency, and note the frequency f_c when V_R just drops to 0.707 V_max.

Frequency response in series RC circuits

Figure 1. Frequency response of a series RC circuit

Capacitors also resist the changing AC voltage, but in an opposite fashion than inductors. The effects of rapidly changing electric fields pass through a capacitor C unimpeded, but now slowly changing fields are "resisted." That means less current at low frequencies. Figure 2 shows the small current values at low frequencies in a series RC circuit.

Just as with the inductor, you can determine the cutoff frequency for the RC circuit. Again, measure V_R across the resistor as you adjust the frequency, and note the frequency f_c when it drops to 0.707 V_max.

Calculating the cutoff frequency

If you know the circuit values for the resistor, capacitor, and inductor, you can calculate the cutoff frequency value.

For the series RL circuit:

For the series RC circuit:

Try these formulas using the circuit values in the figures above and see if your values for f_c agree with the graph. Be careful to include the scientific notation required by the unit prefixes.